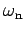
The animated plot on the right shows how the response of an undamped second-order oscillator to a sinusoidal forcing function changes as the frequency of the input sinusoid changes. Each frame of the plot shows the response (in blue) as a function of time on the x axis for a value of input frequency that lies between zero and the natural frequency of the oscillator. The green curve depicts the forcing function itself. The vertical red line depicts the forcing frequency as it increases from 0 to . 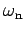Notice that for small values of the forcing frequency, the blue response almost coincides with the green forcing function, that is, the oscillator moves in synchronization with the forcing. This is because the inertia force is small at low frequencies, and the oscillator behaves as if it were only a spring (of unit stiffness for the numbers that were chosen to create the plot). As the forcing frequency increases, the inertia force becomes comparable to the stiffness force, and the response begins to deviate from the forcing function. 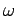The response at any frequency is the superposition of two harmonic oscillations, one at the forcing frequency  and the other at the natural frequency . 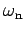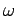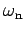As approaches , the response assumes the form of a high frequency oscillation whose amplitude increases and decreases sinusoidally at a relatively lower frequency, and thus exhibits the phenomenon of beats. The last frame of the plot depicts what happens when the forcing frequency equals the natural frequency.  The response is an oscillation whose amplitude grows linearly with time, as indicated by the linear envelope formed by the two straight green lines. This unbounded response is an illustration of the phenomenon of resonance.


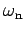
  The animated plot on the left shows the response of a damped second-order oscillator with damping ratio 0.1 to a sinusoidal forcing as the forcing frequency increases from zero to twice the undamped natural frequency . Notice once again, that at low forcing frequencies, the response follows the forcing input very closely, since the inertia and damping forces are weak at low frequencies, and the system essentially behaves like a spring. As in the undamped case, the response is a superposition of a natural response of the system and a forced response. While the forced response is sinusoidal and has the same frequency as the forcing frequency, the natural response in the presence of damping is sinusoidal with an exponentially decaying envelope. Consequently, the natural response is transient and dies out after a few cycles leaving only the forced response in the steady state. The first few cycles of the forcing function in the plot clearly show the transient response, while subsequent cycles show the steady state response. Notice that the steady state response has the same frequency as the forcing function, but differs from the forcing function in amplitude and phase. The plot shows that the steady state response lags the forcing function at all frequencies, and the amount of lag increases with the frequency. On the other hand, the steady state amplitude of achieves a maximum somewhere near resonance, and then steadily decreases as the forcing frequency increases. However, unlike in the undamped case, the response is bounded at all input frequencies. To see how the steady state amplification and phase shift of a second-order system vary with forcing frequency and damping ratio, see frequency response of second-order systems.


The animated plot on the right shows the harmonic response of a lightly damped second-order oscillator having a damping ratio of only 0.02. Qualitatively, the harmonic response of a lightly damped oscillator is not different from that of a moderately damped oscillator such as the one discussed above. However, because of the low damping, the transient response takes a long time to decay. You will notice that the steady state response cannot be seen throughout  the time and frequency range shown on  the plot, indicating that the time scales chosen are not long enough for the transient response to die out. You will also notice the beating phenomenon as well as a large increase in the response amplitude near resonance.